
DA represents a vertical tower.
Points B, A, and C all lie in the same horizontal plane.
The angle of elevation of D at B is x�.
Angles BAC, BAD, and CAD are each 90�.
BD = BC and AD = AC.
Angle DBC is y�.
Prove that cos y = cos2x.

DA represents a vertical tower.
Points B, A, and C all lie in the same horizontal plane.
The angle of elevation of D at B is x�.
Angles BAC, BAD, and CAD are each 90�.
BD = BC and AD = AC.
Angle DBC is y�.
Prove that cos y = cos2x.
MA represents a vertical tower. Points A, B, and C lie in the same horizontal plane. MB and MC are wire stays, each of length 20 meters. The angle of elevation of M at B is x°. ∠MCB is z°, and ∠ABC = y° = ∠ACB.

You are sailing your splendid yacht, the Gemini, down the Hudson River into New York Harbor. It is a sunny afternoon, with a light breeze, and everything is going well. Your crew has been scrubbing down the bulkheads, polishing the brass hardware, and oiling up the teakwood decks. Your chef has begun preparing a splendid dinner on the afterdeck for you and your guests — life is good.
You are approaching the great gray George Washington Bridge, which spans the Hudson from Manhattan Island to New Jersey. Beneath the bridge, on the Manhattan side, is a famous little red lighthouse. You take a sighting of the lighthouse and observe that it is 15 degrees to port. You proceed for two minutes at your stately rate of 5 knots, and you observe that the lighthouse is now 29 degrees to port. How close will you come to the lighthouse as you pass under the bridge?
(Data: 1 knot is approximately 6076 feet per hour.)

APB and AQC are semicircles, the midpoints of whose diameters are M and N, respectively.
MP is perpendicular to AB, and Q is on MP.
The length of BC is 5; the length of QP is 3.
Find the length of the radius of each semicircle.
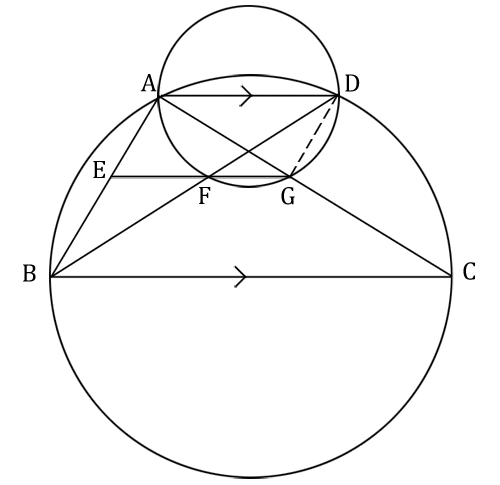
BC is a diameter of circle ADCB. AD is a diameter of circle ADGF.
AD and BC are parallel.
AC and circle ADGF intersect at G; BD and circle ADGF intersect at F.
FG extended meets AB at E.
What kind of shape is ADGE? Prove that your answer is correct.
A father leaves at his death several children who share in his property in the following manner:
Now, it is found in the end that the property has been divided equally among all the children. How much was the fortune, how many children were there, and how much did each child receive?
This problem has been attributed to Sir Isaac Newton. Three cows eat in two weeks all the grass on two acres of land, together with all the grass that grows there in the two weeks. Two cows eat in four weeks all the grass on two acres of land, together with all the grass that grows there in the four weeks. How many cows, then, will eat in six weeks all the grass on six acres of land together with all the grass that grows there in the six weeks? Assume that the quantity of grass on each acre is the same when the cows begin to graze, that the rate of growth is uniform during the time of grazing, and that the cows eat the same amount of grass each week.
A Jetta and a Corvette travel the same distance from Columbus to Cincinnati. The Jetta travels half of the distance at u miles per hour and the other half at v miles per hour. The Corvette travels half of the time at u miles per hour and the other half at v miles per hour. Which car gets to Cincinnati first?
Your science class is on a field trip to a rocket testing site. A rocket sled traveling 900 meters per second on a track passes the place where you are standing. Suddenly, 270 meters farther down the track the rocket explodes quite unexpectedly. If you hear the sound of the explosion 1.1 seconds after the sled passes by, you can calculate the speed of sound. So do it.
Multiply any four consecutive positive integers and add 1 to the product. What kind of a number do you get? Will this always happen? If you think so, prove it.